Geometry/Planetary Information
 |
Constant Values Used [The following value of each constant was used throughout this project. These values are from my notes.]
|
| Newton's Gravitational Constant (G) | 6.6720E-11 |
| Boltzman's Constant (k) | -2.2860E+02 dBW/K/Hz or 1.3800E-23 J/K |
| Speed of Light (c) | 3E+08 m/s |
 |
Neptune Facts and Figures [The following information was used in my calculation and was taken from NASA's National Space Science Data Center.] |
| Mass: | 1.0244E+26 kg |
| Radius: | 2.4764E+04 km |
| Sidereal Day: | 5.7996E+04 seconds |
| Max Distance to Earth: | 4.69E+12 m |
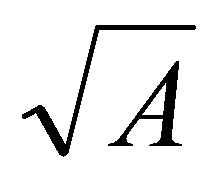
|
Attenuation Next, I solved for the maximum attenuation that could be experienced. This was accomplished by solving for the equatorial probe first. Then I performed some geometric calculations to find the maximum distance through which the +/- 45 degree probes must transmit and adjusted my calculations accordingly. As was done throughout this project, a philosophy was adopted to solve for the worst case scenario, knowing that the design will work under all other conditions, while maximizing robustness. The probes begin at Neptune's gaseous surface and proceed down to a depth of 50 bar (which was experimentally determined to be 420 feet). The raw data was provided as an Excel spreadsheet and was compiled by Georgia Tech researcher Priscilla Mohammed. The expanded Excel file with calculated attenuation curves is included as NeptuneAbsorption.xls |
The data provided was given in 2 km slices, which then had to be accumulated for the primary gases that comprise Neptune's atmosphere. These gases are Hydrogen, Helium, Hydrogen Sulfide, Ammonia, Water, Methane, and Phosphene. Attenuation information was only provided for Water, Ammonia, Hydrogen Sulfide, Phosphene, and Hydrogen. Furthermore, these calculations were performed for 100 MHz, 500 MHz, 1 GHz, and 2.3 GHz.
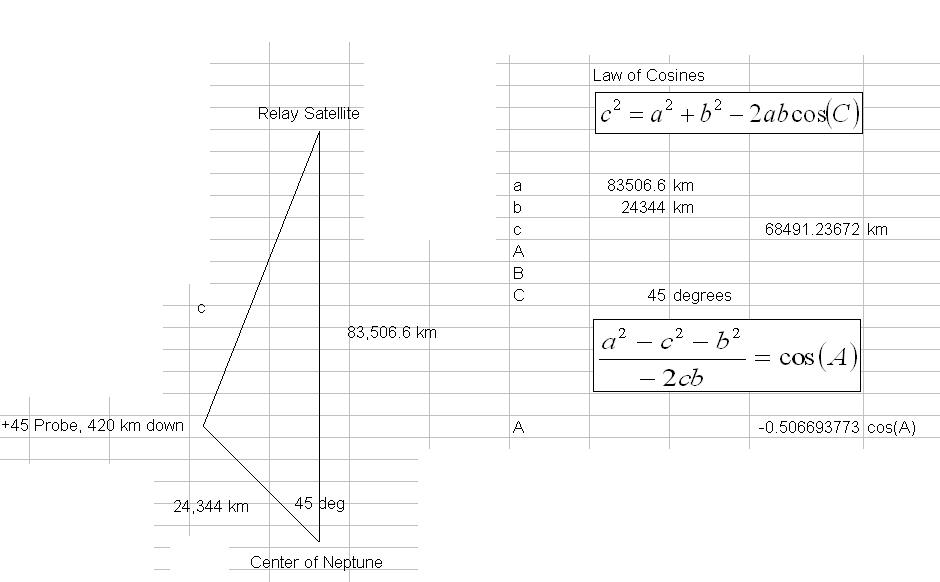
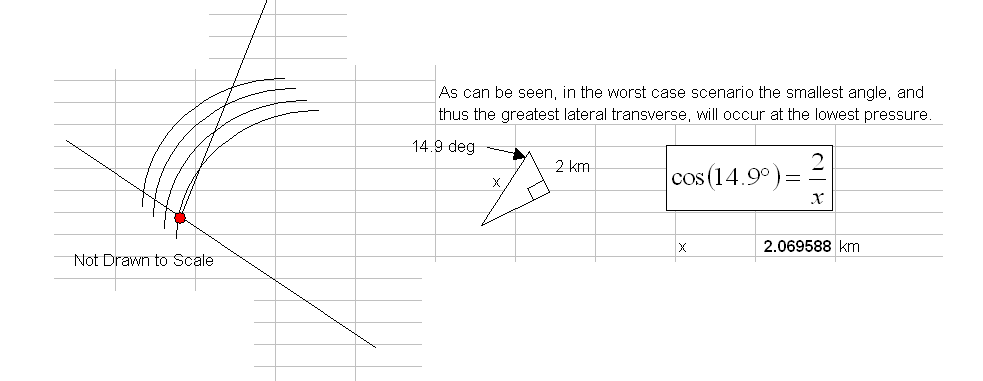
Using geometry and trigonometry, I was able to find the maximum distance transmitted through by the +/- 45 degree probes . These calculations can be found in the included Excel file NepProjCalc.xls.
The following is the resultant attenuation curve for the 45 degree probes.
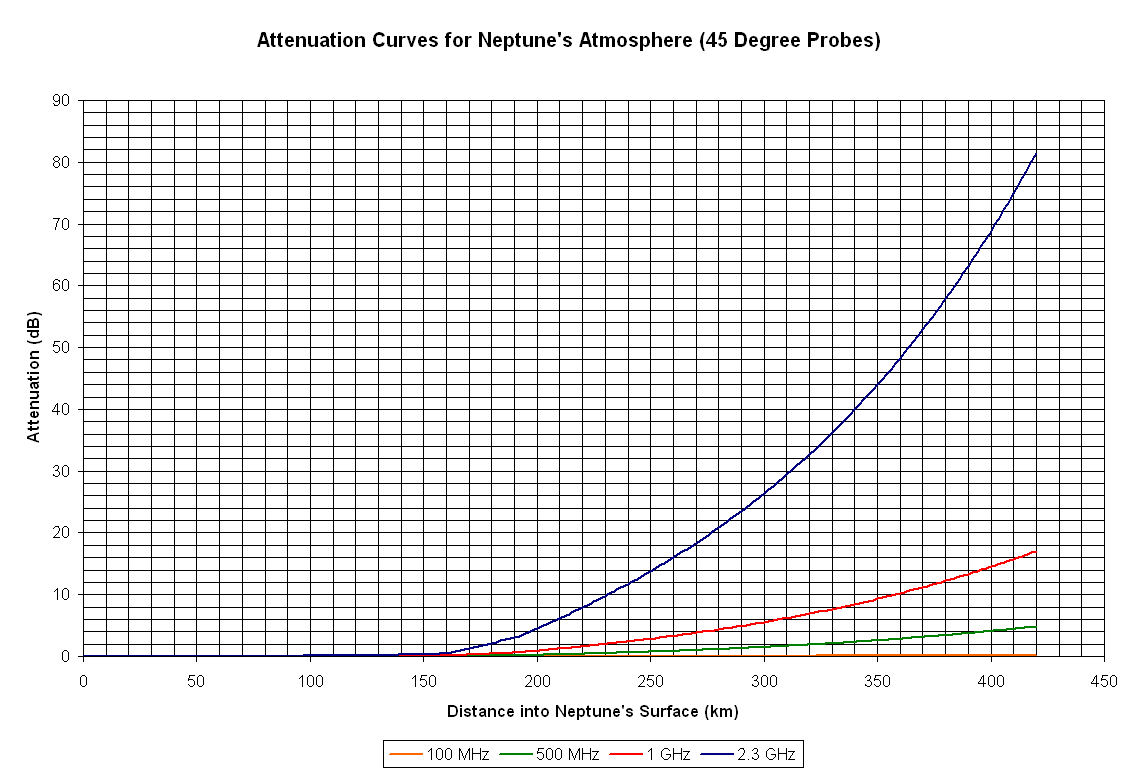
Again viewing the design from a worst-case scenario, I limited my view of the curve to the 420 m case (far right). I wanted to minimize the attenuation I experienced, also keeping in mind that there are no known sources of interference around Neptune. Based upon this logic and a cursory look into communication components, I decided to use a probe transmit frequency of 300 MHz. I then interpolated by using 1/4 the 100 MHz value and 3/4 the 500 MHz value (as the plot is not linear) to find the attenuation at this frequency. Thus, at 50 bar (420 km) below Neptune's surface, the maximum attenuation experienced in a 300 MHz communication link is 3.66 dB.
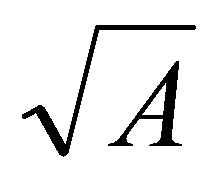
|
Calculating Geostationary Neptunian Orbit Kepler’s third law of planetary motion provides us with the necessary formula for calculating the period of an orbiting body (Pratt 23): T^2=(4π^2a^3)/μ NASA lists one Neptune sidereal day as 16.11 hours, or 57,996 seconds, which we will use as the period, or T. Mu (μ) is equal to Neptune’s mass (1.0244 x 1026 kg) times Newton’s gravitational constant (6.672 x 10-11 Nm2/kg2), or 6.8347968 x 1015. Thus, Neptune’s geostationary orbit radius is 83,506.6 (83,506.5710487) km. |
Geometry - Probes - Relay Satellite - DSN
